

Next: Problem 25
Up: Chapter 32
Previous: Question 2
A beam of particles is directed at a 0.015-kg tumor. There are
 particles per second reaching the tumor,
and the energy of each particle is 4.0 MeV. The RBE for the
radiation is 14. Find the biologically equivalent dose given
to the tumor in 25 s.
particles per second reaching the tumor,
and the energy of each particle is 4.0 MeV. The RBE for the
radiation is 14. Find the biologically equivalent dose given
to the tumor in 25 s.
SOLUTION: The biologically
equivalent dose (BED) is defined as the product of the RBE and the
absorbed dose (AD) in rads. The RBE is a number which characterizes
different types of radiation in terms of their ability to damage
biological material. The AD is defined as the energy
absorbed divided by the mass of the absorbing material. We are given
the RBE. We need to find the AD. We know the mass of the
absorbing material, so we need to find the energy absorbed.
We know the energy per particle, the number of particles per
second, and the total time; the energy absorbed is these three
things multiplied and converted to SI units (grays)
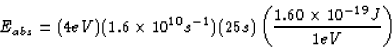
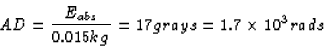
where 1 gray = 100 rads. The BED is then
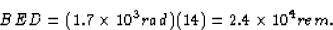
Scott Lanning
4/23/1998
![]()
![]()
![]()