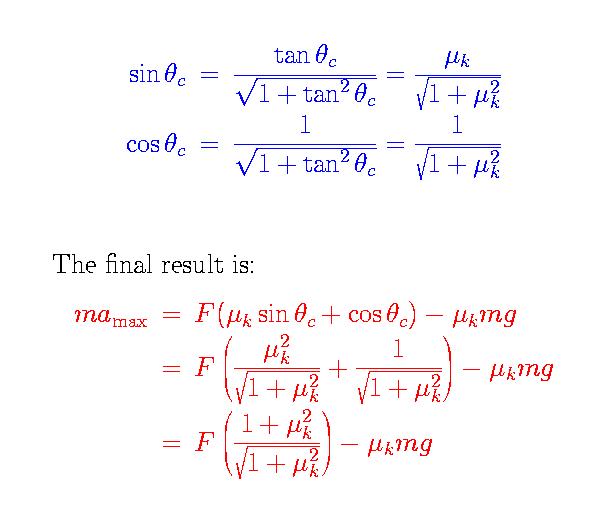
max = Fcosθ - μk(mg - F sin θ) = F(μksin θ + cosθ) - μkmg.
Maximize ax. Compute dax/dθ and set it to zero. This gives: tan θc = μk.
Finally, determine (ax)max by evaluating at the critical angle.
You are pulling a heavy trunk of mass m along a level floor in which the coefficient of sliding is μk. You can pull with a force of fixed magnitude F.
Question: What direction should you pull to accelerate as quickly as possible?
Steps 1-4: See transparency
Step 5: Apply Newton's 2nd law
y-component: F sin θ + N =
mg x-component: F
cosθ - μkN = max
Step 6: Solve
The y equation gives: N = mg - F sin θ.
Substitute into the x equation to give:
max = Fcosθ - μk(mg -
F sin θ) = F(μksin θ + cosθ) -
μkmg.
Maximize ax. Compute dax/dθ and set it to zero.
This gives: tan θc =
μk.
Finally, determine (ax)max by evaluating at the
critical angle.
