Much like the electric field, the electric potential describes the effect of a charge, or set of charges, on the region surrounding it. While the electric field is a vector, the electric potential is a scalar. The electric potential, V, at a given point is the electric potential energy, U, of a small test charge, q, situated at that point divided by the charge itself:
V = U/q
In a uniform field, where U = qEd, the potential V will be given by:
V = Ed
This equation says that every point a distance d away from the chosen zero level has the same electric potential. A set of points that are at the same potential are said to form an equipotential line. In the above simulation, the equipotential lines are horizontal. It is important to note that the equipotential lines are always perpendicular to the field lines. This is true even with non-uniform fields as we will soon see.
Note that the positive charge accelerates toward a region of lower potential and the negative charge accelerates toward a higher potential. In both cases, the potential energy of the charge decreases.
|
|
Click on the milestone icon to answer a conceptual question that will
appear in the milestone window at the upper right. Remember that you can measure V at any point by clicking on that point.
|
The potential from a point charge is:
V = U/q = kQ/r
The equipotential lines here connect all the points with the same r, creating circles centered on the charge. Notice that the circles are perpendicular to the field lines (which you can turn on and off in the simulation).
You might notice that in the above simulation, there is no line marking U = 0 or V = 0. With point charges, the potential is defined to be zero where r is very large or infinite. This is natural considering the form of the potential: when r tends to infinity, 1/r tends to zero.
|
Click on the milestone icon to answer a conceptual question that will
appear in the milestone window at the upper right. Remember that you can measure V at any point by clicking on that point.
|
In Milestone 2, if the test charge is 3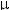 
C, how much work must be done to move it to point B? Assume the value you measure for potential is in Volts.
Like forces and fields, electric potential obeys the principle of superposition. Since electric potential is a scalar, it is much easier to implement because you are adding numbers instead of vectors. At the point where you want to know the net electric potential, you just add the values of electric potential at that point due to the different sources.
Look at this configuration of two unequal charges. Construct the equipotential surfaces and field lines for this configuration by using the buttons below the simulation and double clicking at a point. Which charge is bigger? Notice how the equipotential lines are always perpendicular to the field lines. Also notice that the force vector on the positive test charge always points along the field line.
Answer to question: