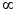
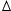V
Consider the parallel-plate capacitor in the above simulation. Change the potential difference between the plates and watch what happens to the charge on the plates. You will notice that:
Q 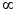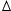V
Or we can write:
Q = C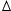V
where C is called the capacitance of the capacitor. The capacitance depends on the geometry of the capacitor and on the material between the plates. Let's explore how the geometry affects the capacitance. We will discuss the effect of materials between the plates later in this Physlab.
Consider a parallel-plate capacitor held at a constant voltage. Since 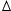V here is constant, Q will now be proportional to C. We will therefore be able to see how C depends on aspects of the geometry by observing Q. Use the simulation to change the distance, d, between the plates and observe what happens to the charge on the plates. You will notice that:
Q 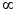1/d
a
Now look at these different sizes for capacitors held at constant voltage. Observe what happens to the charge on the plates when you make the plates larger. You will notice that it seems to be proportional to the length of the plates. It is more accurately proportional to the area, A, of the plate. That is:
Q 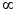A
If we combine these two dependencies, we see that:
Q 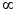A/d
We have determined that the charge here, and therefore the capacitance, is proportional to the area of the capacitor plates and is inversely proportional to the distance between the plates. More completely, for a capacitor with plates separated by a vacuum, capacitance is given by:
C =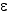 
0A/d
where 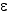0 is the permittivity of free space and has a value of 8.85 x 10-12 C2/Nm2. You have actually used 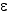0 before without knowing it. It was part of the constant, k, that appeared in Coulomb's law:
k = 1/(4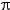
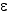0 )
| Capacitor with constant charge -
Click on the milestone icon to answer a conceptual question that will
appear in the milestone window at the upper right.
|
In most capacitors, an electrically insulating material called a dielectric is inserted between the plates. This decreases the electric field inside the capacitor. This happens because the molecules inside the dielectric get polarized in the field and they align themselves in a way that sets up a field in the opposite direction.
You can see how this works in a capacitor with a dielectric by superimposing the fields from a) a capacitor without the dielectric and b) the dielectric. This is easiest to see with field lines. With no dielectric, in the middle picture, the field is about 9 units and this is represented by 9 field lines. The dielectric, in the bottom picture, is polarized by this field and creates a field pointing in the opposite direction that is 6 units. If you put them together, as in the top picture, the field is only 3 units within the dielectric, from the superposition of 9 down and 6 up.
The ratio of the field without the dielectric, E0, and the field with the dielectric, E, is known as the dielectric constant,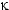 
. That is:
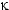= E0/E.
In the case above, the dielectric constant is:
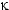= 9/3 = 3.
Note that 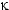is dimensionless and always greater than or equal to one.
For a given potential difference across the plates, a capacitor with a dielectric can store more charge than the one without. The capacitance of a capacitor with a dielectric is given by:
C = 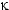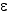0A/d
Look at the magnitude of the electric field for different dielectric constants. What value of 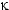corresponds to vacuum?
| Capacitor with constant charge with dielectric-
Click on the milestone icon to answer a conceptual question that will
appear in the milestone window at the upper right.
|
The energy stored in a capacitor is the same as the work it takes to charge the capacitor. Charging a capacitor generally means moving electrons from the positive plate to the negative plate. As the net charge on the plates increases, it gets harder to move the electrons. For each electron, the work required to move it is the its charge multiplied by the potential difference it moves through. As the charge on the plates increases, the potential difference. If the potential difference between the two plates is V at the end of the process, and zero at the start, the average potential difference through which the electrons have moved is V/2. Multiplying this average potential difference by the total charge moved gives the potential energy stored in the capacitor: U = (1/2)QV.
You can see how this works in this simulation. It takes 2 units of work to move the first charge but 4 units to move the second charge (after the second charge is moved, the total work is 6). It then takes 6 units to move the third charge, 8 to move the fourth and 10 to move the last one, for a total of 30.
So the energy stored in the capacitor is W = U = (1/2)QV
30 = (1/2)(5)V
If we solve for V, we get the ending potential difference: V = 12. The average potential difference is V/2 = 6. Note that this corresponds numerically to the work required to move the third (middle) charge. This is because each charge was taken to be one unit. Multiplying the number of charges moved, 5, by 6, the work done on average to move a charge gives 30, the total energy stored in the capacitor.