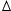V/d where
In previous Physlabs we discussed uniform electric fields. How are these uniform fields produced? One way is with a parallel-plate capacitor: two parallel metal plates placed near one another. A charge +q is placed on one plate while a charge -q is placed on the other plate. In the region between the plates and away from the edges, the electric field, pointing from the positive plate to the negative plate, is uniform.
For a capacitor with infinitely large plates, the value of the constant electric field that it produces is:
E = 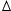V/d where
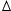V is the potential difference between the plates
In the above simulation, the value of the potential difference, 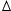V, is +5V - (-5V) = 10V. It is this value, 10 Volts, that determines the electric field along with the distance between the plates. The bottom plate could be labeled V = 0 V and the top plate at V = 10 V and the same electric field would result.
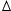Use the simulation to verify the equation E = V/d by moving the plates and measuring E at different values of d. What happens to the charge distribution as you bring the plates closer together?
| What will increase its kinetic energy?
Click on the milestone icon to answer a conceptual question that will
appear in the milestone window at the upper right. Recall that the potential energy of a charge, q, sitting at a potential, V, is given by: U = qV.
|
If we replace the ion in Milestone 1 with an electron with charge -1.6 x 10-19 C and mass 9.1 x 10-31 kg, how fast is it going when it hits the positive plate? Assume the voltage you measure is in Volts.
Since equipotential lines are always perpendicular to field lines, the equipotentials for the parallel plate capacitor must lie parallel to the plates. Consider the bottom plate where V = -5 V. The plate itself is an equipotential surface: the whole thing is at the same potential of -5 V. If you move perpendicular to the plates, toward the top of the simulation, you will be moving to another equipotential surface, say at V = -2.5 V. It is very similar to climbing stairs. If you move up or down the stairs you increase or decrease your potential energy but if you move horizontally along a stair you do not change your potential energy.
In the above simulations the length of each plate is much larger than the separation between them. What happens if the plates are not so large? Look at these four different plate sizes. Notice the charge distribution on each. When the plates are very small, the charge is distributed all around the outside. As the plates get larger, they "notice" the oppositely charged plate more and the charges start to gather on the side closer to the other plate. The more the charges gather there, the closer the field gets to being uniform.
When the ratio of plate length to plate separation gets too small, i.e. the plates are not long enough and/or the distance is too large, you will notice that the field is not uniform, particularly at the edges. This is called a fringe field.
Answer to question: